Welcome to technical page
CLT with MATLAB |
Invariant theory |
Marine turbine |
Statistics <= |
Fibre packing
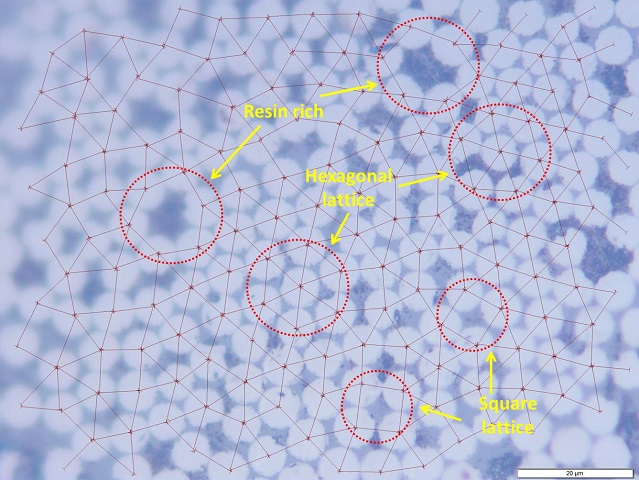
There are several fibre packing assumptions to predict the fibre volume fraction, such as hexagonal and square lattices. Theoretically, it is predicted that the maximum fibre volume fraction is 90.7% for hexagonal lattice and 78.5% for a square lattice.
To observe the fibre packing, an optical image of the microstructure should taken in advance. The specimens were set in a mould and encapsulated by transparent resin, and then ground and polished to be optically flat using a suspension containing 1.5μm diamond particles. The mould looks like this,

The microscopic images were taken from an optical microscope using 100 times optical zoom associating with 10 times electronic zoom (OLYMPUS BX60M), and the distances were measured by OLYMPUS STREAM software (version 1.9). A mixture of the hexagonal and square lattices can be seen, with quite a few resin rich volumes,
The measurements of the distances were then imported into MATLAB for the fitting using DFITTOOL toolbox,
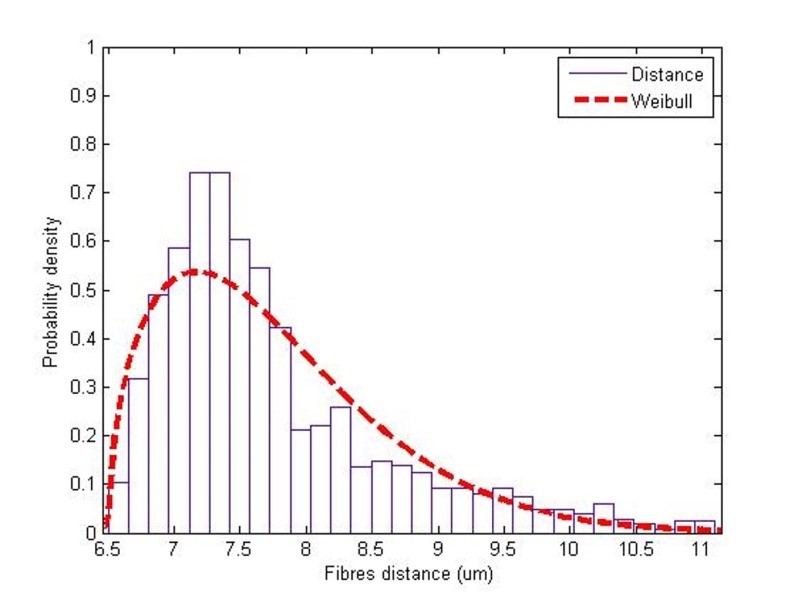
The Weibull fitting can be presented as,
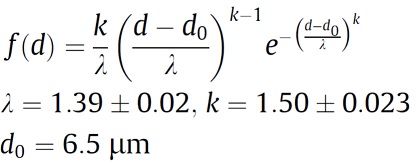
For more information, please read the published paper (link to ScienceDirect)
Fibre misalignment
The misalignment angle can be obtained by an indirect approach, which requires to measure the ratio of short/long radius of the projective ellipse.
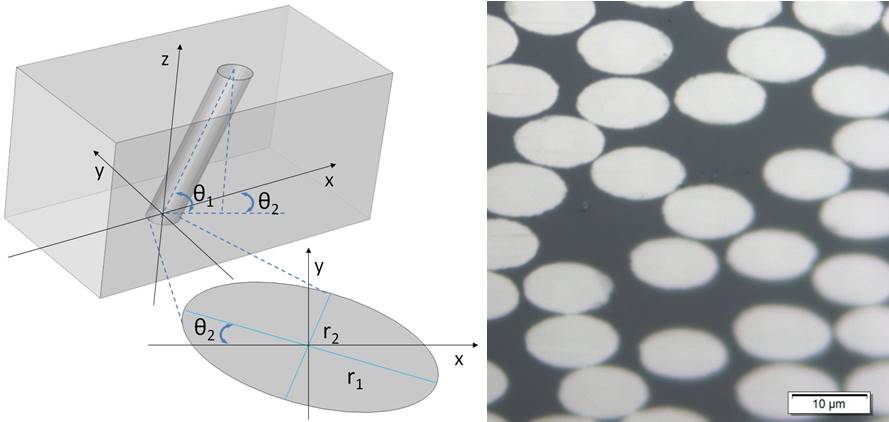
Thousands of ellipses were measured by hand (very intensive work indeed). Luckly, the OLYMPUS STREAM software recorded the individial radius, so that these data were imported into MATLAB and were fitted by DFITTOOL toolbox again. The probobility density looks like this (Gaussion distribution),

The Gaussion fitting (normal fit) can be expressed as,
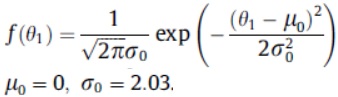
For more information, please read the published paper (link to ScienceDirect)
